Geometry: Let's Get Parallel

Let's Get Parallel

You were introduced to Euclid's Parallel Postulate. That postulate has raised more questions in the last 2,000 years than any other postulate. Euclid spent a lot of time pondering parallel lines. He was very uncomfortable with his 5th postulate, because he firmly believed that he could prove it from other postulates and theorems. In this section, you'll be dealing with pairs of lines cut by a transversal. Remember all of the angle relationships that were involved with lines and transversals. There are alternate interior and exterior angles, same-side interior and exterior angles, corresponding angles, and vertical angles. Feel free to review these topics if you need some refreshment.

When dealing with parallel lines, you can freely invoke Euclid's 5th postulate. It's important to keep in mind that many of these results only hold for Euclidean geometry. You'll have to wait until Whose Geometry Is It Anyway? to enter the bizarre world of non-Euclidean geometry.

In order to explore the relationships between angles formed when parallel lines are cut by a transversal, you need a place to start. It's time to add another postulate to your collection.

• Postulate 10.1: If two parallel lines are cut by a transversal, then the corresponding angles are congruent.

To get a feel for this theorem, take a look at Figure 10.3. If lines l and m are parallel, and line t is a transversal, then ˆ 1 and ˆ 2 are corresponding angles. According to Postulate 10.1, ˆ 1 ~= ˆ 2. We can use this postulate to solve €œfind the measure of the angle€ puzzles.

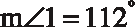
Figure 10.3Lines l and m are parallel, line t is a transversal.

• Example 2: In Figure 10.4, l €Œ €Œ m and mˆ 1 = 112º. Find mˆ 2, mˆ 4, mˆ 5, and mˆ 8.

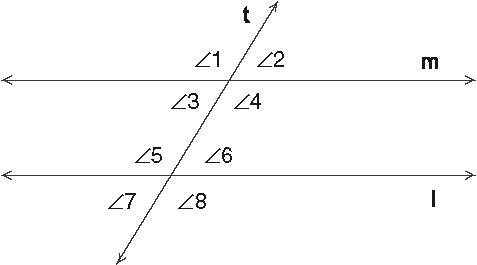
Figure 10.4l €Œ €Œ m and mˆ 2 = 112º.

• Solution: Use Postulate 10.1, and the theorem about vertical angles to solve this problem. Because ˆ 1 and ˆ 4 are vertical angles, you know that they are congruent. Right away you know that mˆ 4 = 112º. Because ˆ 4 and ˆ 8 are corresponding angles, by Postulate 10.1 you know that ˆ 4 and ˆ 8. Because mˆ 4 = 112º , mˆ 8 =112 º. Because ˆ 8 and ˆ 5 are vertical angles, you know that ˆ 5 ~= ˆ 8, so mˆ 5 112º. All that's left is to find mˆ 2. Because ˆ 1 and ˆ 2 are supplementary angles (together they form a straight angle), you know that mˆ 1 + mˆ 2 = 180º. Substituting in for mˆ 1, you see that mˆ 1 + 112º = 180º. So mˆ 2 = 68º. To summarize: mˆ 2 = 68º , mˆ 4 = 112º , ˆ 5 = 112º , and mˆ 8 = 112º.

Excerpted from The Complete Idiot's Guide to Geometry © 2004 by Denise Szecsei, Ph.D.. All rights reserved including the right of reproduction in whole or in part in any form. Used by arrangement with Alpha Books, a member of Penguin Group (USA) Inc.

To order this book direct from the publisher, visit the Penguin USA website or call 1-800-253-6476. You can also purchase this book at Amazon.com and Barnes & Noble.