Geometry: Cylinders and Cones
Cylinders and Cones
In the definition of a prism, the bases were forced to be congruent polygons. If the bases are congruent closed curves (like a circle, for example) then you have a cylinder. I've sketched a cylinder with a circular base in Figure 21.4. The base of this cylinder is a circle so it doesn't have any vertices. Most of the cylinders you encounter have circles for the shape of the base, and they are called circular cylinders. But having a circular base is just a courtesy. It is not a requirement.
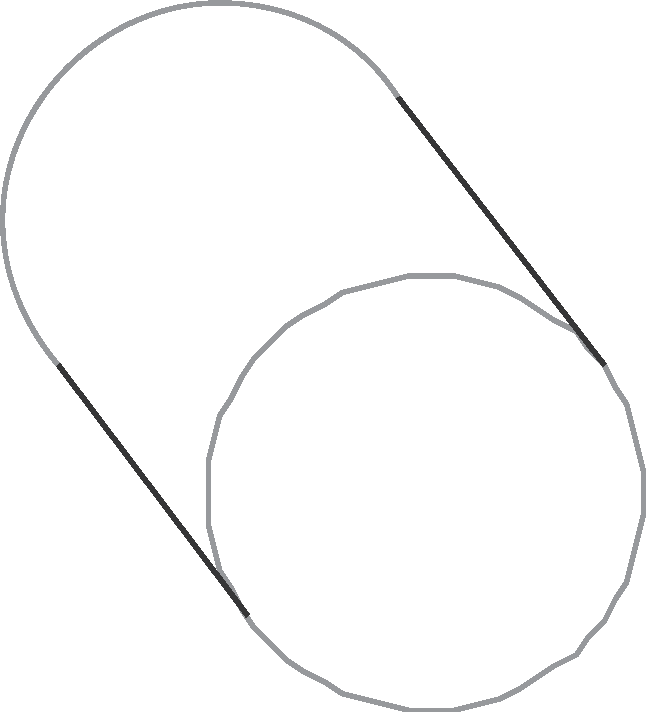
Figure 21.4A circular cylinder.
When you think of a cylinder, the immediate shape that comes to mind is that of a tin can. These types of cylinders are classified as right circular cylinders.
Loosening up the restrictions on the bases of a prism created cylinders. Loosening up the restrictions on the shape of the base of a pyramid will result in the creation of a cone. Construct a cone the same way you constructed a pyramid. Start with a closed curve lying in a plane, which will serve as the base of the cone, and a point, which will serve as the apex of the cone. A cone is the solid formed by the interior of this closed curve together with the surface formed when each point on the closed curve is joined to the apex using line segments.
Solid Facts
A cylinder is the solid generated by two congruent closed curves in parallel planes together with the surface formed by line segments joining corresponding points of the two curves. A circular cylinder is a cylinder with a circular base.
A cone is named based on the shape of its base. Figure 21.5 shows a circular cone. Circular cones fall into one of two categories: right circular cones and oblique circular cones. A right circular cone is a circular cone where the line segment connecting the apex of the cone to the center of the circular base is perpendicular to the plane of the base. An oblique circular cone is a circular cone where the line segment connecting the apex of the cone to the center of the circular base is not perpendicular to the plane of the base.

Figure 21.5A circular cone.
Solid Facts
A cone is the solid formed by the interior of a planar closed curve together with the surface formed when each point on the closed curve is joined to a noncoplanar point using line segments.
The base of a cone is the planar closed curve of the cone.
The apex of a cone is the noncoplanar point that is joined to every point on the base.
A right circular cone is a circular cone where the line segment connecting the apex of the cone to the center of the circular base is perpendicular to the plane of the base.
An oblique circular cone is a circular cone where the line segment connecting the apex of the cone to the center of the circular base is not perpendicular to the plane of the base.
If you are having trouble visualizing the mathematical description of a cone, you can find a model of one at your favorite ice cream shop. Ask for your favorite flavor in the traditional serving container, a “sugar” cone. This is just a model of a cone, and some slight differences between the model and the real thing are bound to exist. For example, a mathematician's cone is solid, while the ice-cream cone is hollow. But you can avoid this difference by filling the cone with ice cream.
Excerpted from The Complete Idiot's Guide to Geometry © 2004 by Denise Szecsei, Ph.D.. All rights reserved including the right of reproduction in whole or in part in any form. Used by arrangement with Alpha Books, a member of Penguin Group (USA) Inc.
To order this book direct from the publisher, visit the Penguin USA website or call 1-800-253-6476. You can also purchase this book at Amazon.com and Barnes & Noble.







