Geometry: Answer Key

Answer Key

Geometry

  • Answer Key

This provides the answers and solutions for the Put Me in, Coach! exercise boxes, organized by sections.

Taking the Burden out of Proofs

  1. Yes
  2. Theorem 8.3: If two angles are complementary to the same angle, then these two angles are congruent.

A and B are complementary, and C and B are complementary.

Given: A and B are complementary, and C and B are complementary.

Prove: A ~= C.

  Statements Reasons
1. A and B are complementary, and C and B are complementary. Given
2. mA + mB = 90º , mC + mB = 90º Definition of complementary
3. mA = 90 º - mB, mC = 90º - mB Subtraction property of equality
4. mA = mC Substitution (step 3)
5. A ~= C Definition of ~=

Proving Segment and Angle Relationships

  1. If E is between D and F, then DE = DF EF.
E is between D and F.

E is between D and F.

Given: E is between D and F

Prove: DE = DF EF.

  Statements Reasons
1. E is between D and F Given
2. D, E, and F are collinear points, and E is on ¯DF Definition of between
3. DE + EF = DF Segment Addition Postulate
4. DE = DF EF Subtraction property of equality

2. If BD divides ABC into two angles, ABD and DBC, then mABC = mABC - mDBC.

BD divides ABC into two angles, ABD and DBC.

Given: BD divides ABC into two angles, ABD and DBC

Prove: mABD = mABC - mDBC.

Reasons
  Statements
1. BD divides ABC into two angles, ABD and DBC Given
2. mABD + mDBC = mABC Angle Addition Postulate
3. mABD = mABC - mDBC Subtraction property of equality

3. The angle bisector of an angle is unique.

ABC with two angle bisectors: BD and BE.

Given: ABC with two angle bisectors: BD and BE.

Prove: mDBC = 0.

  Statements Reasons
1. BD and BE bisect ABC Given
2. ABC ~= DBC and ABE ~= EBC Definition of angel bisector
3. mABD = mDBC and mABE ~= mEBC Definition of ~=
4. mABD + mDBE + mEBC = mABC Angle Addition Postulate
5. mABD + mDBC = mABC and mABE + mEBC = mABC Angle Addition Postulate
6. 2mABD = mABC and 2mEBC = mABC Substitution (steps 3 and 5)
7. mABD = mABC/2 and mEBC = mABC/2 Algebra
8. mABC/2 + mDBE + mABC/2 = mABC Substitution (steps 4 and 7)
9. mABC + mDBE = mABC Algebra
10. mDBE = 0 Subtraction property of equality

4. The supplement of a right angle is a right angle.

A and B are supplementary angles, and A is a right angle.

Given: A and B are supplementary angles, and A is a right angle.

Prove: B is a right angle.

  Statements Reasons
1. A and B are supplementary angles, and A is a right angle Given
2. mA + mB = 180º Definition of supplementary angles
3. mA = 90º Definition of right angle
4. 90º + mB = 180º Substitution (steps 2 and 3)
5. mB = 90º Algebra
6. B is a right angle Definition of right angle

Proving Relationships Between Lines

  1. m6 = 105º , m8 = 75º
  2. Theorem 10.3: If two parallel lines are cut by a transversal, then the alternate exterior angles are congruent.

l m cut by a transversal t.

Given: l m cut by a transversal t.

Prove: 1 ~= 3.

  Statements Reasons
1. l m cut by a transversal t Given
2. 1 and 2 are vertical angles Definition of vertical angles
3. 2 and 3 are corresponding angles Definition of corresponding angles
4. 2 ~= 3 Postulate 10.1
5. 1 ~= 2 Theorem 8.1
6. 1 ~= 3 Transitive property of 3.

3. Theorem 10.5: If two parallel lines are cut by a transversal, then the exterior angles on the same side of the transversal are supplementary angles.

l m cut by a transversal t.

Given: l m cut by a transversal t.

Prove: 1 and 3 are supplementary.

  Statement Reasons
1. l m cut by a transversal t Given
2. 1 and 2 are supplementary angles, and m1 + m2 = 180º Definition of supplementary angles
3. 2 and 3 are corresponding angles Definition of corresponding angles
4. 2 ~= 3 Postulate 10.1
5. m2 ~= m3 Definition of ~=
6. m1 + m3 = 180º Substitution (steps 2 and 5)
7. 1 and 3 are supplementary Definition of supplementary

 

The Top 3D Printers
3D Printers

Interested in 3D Printing?

We’ve researched the top things you should consider when purchasing a 3D printer, and have chosen the best printers of 2020 based on your needs.

GET PRINTING

 

4. Theorem 10.9: If two lines are cut by a transversal so that the alternate exterior angles are congruent, then these lines are parallel.

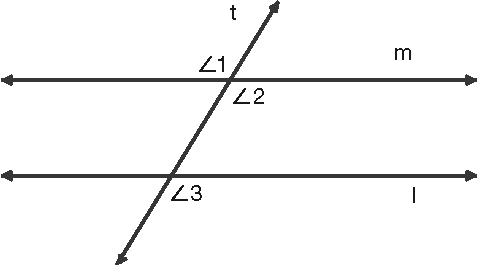
Lines l and m are cut by a transversal t.

Lines l and m are cut by a transversal t.

Given: Lines l and m are cut by a transversal t, with 1 ~= 3.

Prove: l m.

  Statement Reasons
1. Lines l and m are cut by a transversal t, with 1 ~= 3 Given
2. 1 and 2 are vertical angles Definition of vertical angles
3. 1 ~= 2 Theorem 8.1
4. 2 ~= 3 Transitive property of ~=.
5. 2 and 3 are corresponding angles Definition of corresponding angles
6. l m Theorem 10.7

5. Theorem 10.11: If two lines are cut by a transversal so that the exterior angles on the same side of the transversal are supplementary, then these lines are parallel.

Lines l and m are cut by a t transversal t.

Lines l and m are cut by a t transversal t.

Given: Lines l and m are cut by a transversal t, 1 and 3 are supplementary angles.

Prove: l m.

  Statement Reasons
1. Lines l and m are cut by a transversal t, and 1 are 3 supplementary angles Given
2. 2 and 1 are supplementary angles Definition of supplementary angles
3. 3 ~= 2 Example 2
4. 3 and 2 are corresponding angles Definition of corresponding angles
5. l m Theorem 10.7

Two's Company. Three's a Triangle

  1. An isosceles obtuse triangle
  2. The acute angles of a right triangle are complementary.

ABC is a right triangle.

Given: ABC is a right triangle, and B is a right angle.

Prove: A and C are complementary angles.

  Statement Reasons
1. ABC is a right triangle, and B is a right angle Given
2. mB = 90º Definition of right angle
3. mA + mB + mC = 180º Theorem 11.1
4. mA + 90º + mC = 180º Substitution (steps 2 and 3)
5. mA + mC = 90º Algebra
6. A and C are complementary angles Definition of complementary angles

3. Theorem 11.3: The measure of an exterior angle of a triangle equals the sum of the measures of the two nonadjacent interior angles.

ABC with exterior angle BCD.

  Statement Reasons
1. ABC with exterior angle BCD Given
2. DCA is a straight angle, and mDCA = 180º Definition of straight angle
3. mBCA + mBCD = mDCA Angle Addition Postulate
4. mBCA + mBCD = 180º Substitution (steps 2 and 3)
5. mBAC + mABC + mBCA = 180º Theorem 11.1
6. mBAC + mABC + mBCA = mBCA + mBCD Substitution (steps 4 and 5)
7. mBAC + mABC = mBCD Subtraction property of equality

4. 12 units2

5. 30 units2

6. No, a triangle with these side lengths would violate the triangle inequality.

Congruent Triangles

1. Reflexive property: ABC ~= ABC.

Symmetric property: If ABC ~= DEF, then DEF ~= ABC.

Transitive property: If ABC ~= DEF and DEF ~= RST, then ABC ~= RST.

2. Proof: If ¯AC ~= ¯CD and ACB ~= DCB as shown in Figure 12.5, then ACB ~= DCB.

  Statement Reasons
1. ¯AC ~= ¯CD and ACB ~= DCB Given
2. ¯BC ~= ¯BC Reflexive property of ~=
3. ACB ~= DCB SAS Postulate

3. If ¯CB ¯AD and ACB ~= DCB, as shown in Figure 12.8, then ACB ~= DCB.

  Statement Reasons
1. ¯CB ¯AD and ACB ~= DCB Given
2. ABC and DBC are right angles Definition of
3. mABC = 90º and mDBC = 90º Definition of right angles
4. mABC = mDBC Substitution (step 3)
5. ABC ~= DBC Definition of ~=
6. ¯BC ~= ¯BC Reflexive property of ~=
7. ACB ~= DCB ASA Postulate

4. If ¯CB ¯AD and CAB ~= CDB, as shown in Figure 12.10, then ACB~= DCB.

  Statement Reasons
1. ¯CB ¯AD and CAB ~= CDB Given
2. ABC and DBC are right angles Definition of
3. mABC = 90º and mDBC = 90º Definition of right angles
4. mABC = mDBC Substitution (step 3)
5. ABC ~= DBC Definition of ~=
6. ¯BC ~= ¯BC Reflexive property of ~=
7. ACB ~= DCB AAS Theorem

5. If ¯CB ¯AD and ¯AC ~= ¯CD, as shown in Figure 12.12, then ACB ~= DCB.

  Statement Reasons
1. ¯CB ¯AD and ¯AC ~= ¯CD Given
2. ABC and DBC are right triangles Definition of right triangle
3. ¯BC ~= ¯BC Reflexive property of ~=
4. ACB ~= DCB HL Theorem for right triangles

6. If P ~= R and M is the midpoint of ¯PR, as shown in Figure 12.17, then N ~= Q.

  Statement Reasons
1. P ~= R and M is the midpoint of ¯PR Given
2. ¯PM ~= ¯MR Definition of midpoint
3. NMP and RMQ are vertical angles Definition of vertical angles
4. NMP ~= RMQ Theorem 8.1
5. PMN ~= RMQ ASA Postulate
6. N ~= Q CPOCTAC

Smiliar Triangles

  1. x = 11
  2. x = 12
  3. 40º and 140º
  4. If A ~= D as shown in Figure 13.6, then BC/AB = CE/DE.
  Statement Reasons
1. A ~= D Given
2. BCA and DCE are vertical angles Definition of vertical angles
3. BCA ~= DCE Theorem 8.1
4. ACB ~ DCE AA Similarity Theorem
5. BC/AB = CE/DE CSSTAP

5. 150 feet.

Opening Doors with Similar Triangles

  1. If a line is parallel to one side of a triangle and passes through the midpoint of a second side, then it will pass through the midpoint of the third side.

¯DE ¯AC and D is the midpoint of ¯AB.

Given: ¯DE ¯AC and D is the midpoint of ¯AB.

Prove: E is the midpoint of ¯BC.

  Statement Reasons
1. ¯DE ¯AC and D is the midpoint of ¯AB. Given
2. ¯DE ¯AC and is cut by transversal AB Definition of transversal
3. BDE and BAC are corresponding angles Definition of corresponding angles
4. BDE ~= BAC Postulate 10.1
5. B ~=B Reflexive property of ~=
6. ABC ~ DBE AA Similarity Theorem
7. DB/AB = BE/BC CSSTAP
8. DB = AB/2 Theorem 9.1
9. DB/AB = 1/2 Algebra
10. 1/2 = BE/BC Substitution (steps 7 and 9)
11. BC = 2BE Algebra
12. BE + EC = BC Segment Addition Postulate
13. BE + EC = 2BE Substitution (steps 11 and 12)
14. EC = BE Algebra
15. E is the midpoint of ¯BC Definition of midpoint

2. AC = 43 , AB = 8 , RS = 16, RT = 83

3. AC = 42 , BC = 42

Putting Quadrilaterals in the Forefront

  1. AD = 63, BC = 27, RS = 45
  2. ¯AX, ¯CZ, and ¯DY
Trapezoid ABCD with its XB CY four altitudes shown.

Trapezoid ABCD with its XB CY four altitudes shown.

3. Theorem 15.5: In a kite, one pair of opposite angles is congruent.

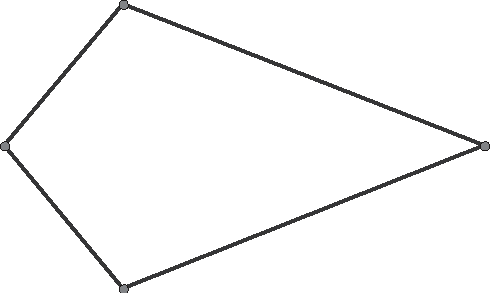
Kite ABCD.

Kite ABCD.

Given: Kite ABCD.

Prove: B ~= D.

  Statement Reasons
1. ABCD is a kite Given
2. ¯AB ~= ¯AD and ¯BC ~= ¯DC Definition of a kite
3. ¯AC ~= ¯AC Reflexive property of ~=
4. ABC ~= ADC SSS Postulate
5. B ~= D CPOCTAC

4. Theorem 15.6: The diagonals of a kite are perpendicular, and the diagonal opposite the congruent angles bisects the other diagonal.

Kite ABCD.

Kite ABCD.

Given: Kite ABCD.

Prove: ¯BD ¯AC and ¯BM ~= ¯MD.

  Statement Reasons
1. ABCD is a kite Given
2. ¯AB ~= ¯AD and ¯BC ~= ¯DC Definition of a kite
3. ¯AC ~= ¯AC Reflexive property of ~=
4. ABC ~= ADC SSS Postulate
5. BAC ~= DAC CPOCTAC
6. ¯AM ~= ¯AM Reflexive property of ~=
7. ABM ~= ADM SAS Postulate
8. ¯BM ~= ¯MD CPOCTAC
9. BMA ~= DMA CPOCTAC
10. mBMA = mDMA Definition of ~=
11. MBD is a straight angle, and mBMD = 180º Definition of straight angle
12. mBMA + mDMA = mBMD Angle Addition Postulate
13. mBMA + mDMA = 180º Substitution (steps 9 and 10)
14. 2mBMA = 180º Substitution (steps 9 and 12)
15. mBMA = 90º Algebra
16. BMA is a right angle Definition of right angle
17. ¯BD ¯AC Definition of

5. Theorem 15.9: Opposite angles of a parallelogram are congruent.

Parallelogram ABCD.

Given: Parallelogram ABCD.

Prove: ABC ~= ADC.

  Statement Reasons
1. Parallelogram ABCD has diagonal ¯AC. Given
2. ABC ~= CDA Theorem 15.7
3. ABC ~= ADC CPOCTAC

6. 144 units2

7. 180 units2

8. Kite ABCD has area 48 units2.

Parallelogram ABCD has area 150 units2.

Rectangle ABCD has area 104 units2.

Rhombus ABCD has area 35/2 units2.

Anatomy of a Circle

  1. Circumference: 20 feet, length of ˆRST = 155/18 feet
  2. 9 feet2
  3. 15 feet2
  4. 28º

The Unit Circle and Trigonometry

  1. 3/34 = 334/34
  2. 1/3 = 3/3
  3. tangent ratio = 40/3, sine ratio = 40/7
  4. tangent ratio = 5/56 = 556/56, cosine ratio = 56/9

Excerpted from The Complete Idiot's Guide to Geometry © 2004 by Denise Szecsei, Ph.D.. All rights reserved including the right of reproduction in whole or in part in any form. Used by arrangement with Alpha Books, a member of Penguin Group (USA) Inc.

To order this book direct from the publisher, visit the Penguin USA website or call 1-800-253-6476. You can also purchase this book at Amazon.com and Barnes & Noble.

 
 

 

sandbbox logo
©2022 Sandbox Networks Inc. All rights reserved. Sandbox Learning is part of Sandbox & Co., a digital learning company.